I’ve decided to try something new: once a week, to write a shorter, narrower post focusing on one specific paper. Last week, I wrote about discrimination against gay couples in the housing market. This is the tab with all the previous (and current) posts.
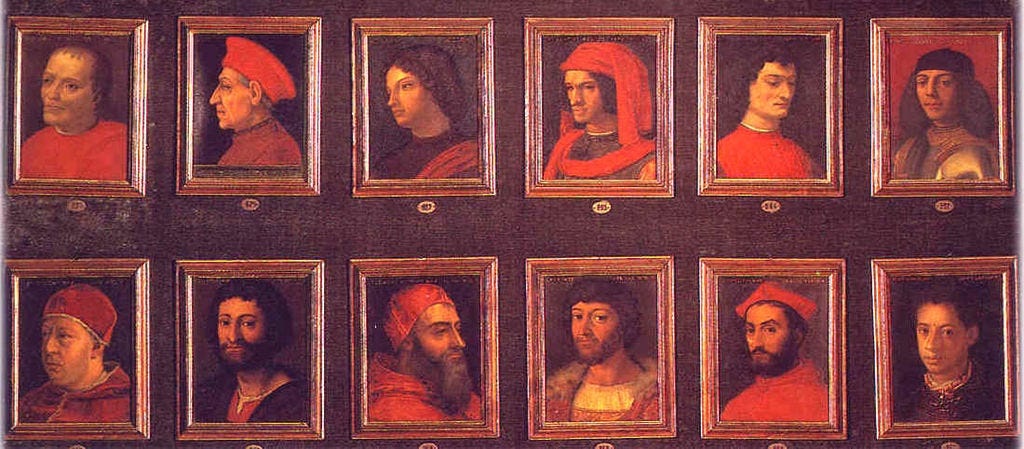
Onto the actual post: are families that used to be wealthy in the 15th century more likely to be wealthy today?
“Her voice is full of money,” he said suddenly.
That was it. I’d never understood before. It was full of money–that was the inexhaustible charm that rose and fell in it, the jingle of it, the cymbals’ song of it.F. Scott Fitzgerald, “The Great Gatsby”
As I’ve written before, socioeconomic mobility - i.e. the ability of someone born in a poor background to end up rich, or vice versa - is on the decline. Rich people are remaining rich, while nobody else catches up. How long has this been going on? Well, according to Gugliemo Barone and Sauro Mocetti’s paper “Intergenerational Mobility in the Very Long Run: Florence 1427–2011” (ungated version here), it’s been around 500 years.
The core concept to define is socioeconomic mobility: the capacity of someone from a given part of the income or wealth distribution to be able to move up (or down) “on their own”, so to speak. In the US, socioeconomic mobility is low, has been falling for decades, and is lower for non-whites than whites. In fact, few people are socioeconomically mobile: it’s very rare for the children of the poorest Americans to become rich, or the inverse, and most people remain at the income level they were born with, give or take a few percentiles of the income distribution.
A very important thing to note is what “percentile” or “decile” means: if you arrange everyone by income, from lowest to highest (the “income distribution”, so to speak), a percentile is a group of people of 1%, and the “fifteenth percentile” is the group at the 15% mark - while the 85th percentile is the group at the 85% mark, aka the top 15% (this gets complicated quickly). A decile is the same thing, except arranged in 10% intervals - so the fourth decile is the 40% mark; and a quintile is a group of 20% of the population. There’s also quirked up ones, like a tertile (33%) or sextile (16.7%).
The paper has a relatively simple methodology: the Italian city of Florence, which was a hub of the Renaissance Era, levied a tax on most of its population in 1427, so that they could fund one of its ongoing wars with Milan. To collect this new tax, the Florentines ordered a census, and the city later published data for taxpayers in 1427. This included surnames, occupations, earnings and (most importantly) wealth. In the present day (2005 and 2011), the Italian Revenue Service provided individualized datasets, per last name, of taxpayers, alongside their income (of various types), occupation, age, gender, and whatnot.
One first item to note is that Italian surnames, like in most Western countries, are inherited patrilinneally, meaning that fathers pass them on to their sons. The surnames have a variety of origins: patronymics, such as Mattei and Di Matteo, which “of Matteo” (aka of Matthew); professions - Medici means “doctors”, Martelli means “hammers” (smiths), Forni means “oven” (cooks); locations, such as Romano ("Roman”), or Piazza (“Square”); or nicknames - like Basso (“short”) or Grasso (“fat”). Because some last names also have specific qualities based on their region of origin (for example, the Veneto adds “n” to last names, case in point Benetton), Italy has a large number of surnames, such that the 100 most common last names only account for 7% of the population, compared to 22% in England or 17% in the United States1. In addition, names are highly regionalized in Italy, which means that a lot of last names are unique to each region - there is barely any correlation between the Florentine naming distribution and the Italian-at-large distribution.
The Renaissance era surnames were latter associated with their descendants living in 2011. This was done through two steps of simple regressions: the first, of income in the year of interest (1427 and 2011), controlling for a series of relevant variables (age, occupation, gender, etc.) and a series of dummies for each last name so you can compare the Medicis, or whomever, to their descendants. This allows to calculate, thus, the probability of someone with the last name Medici of being wealthy in 1427, and the probability of someone with said last name to be wealthy in 2011. Each last name is weighed by its frequency, too.
As an additional test, a similar regression was included to test for the persistence of professions such as lawyer, banker, doctor, goldsmith, or pharmacist, which are both long-lasting and wealthy. Merging the two datasets, this allowed for an estimate of the probability that a given person with a given last name in a given year have the same profession of a person with the same last name in the other year - so one Mr Medici, banker, 2011, having an ancestor who was Mr Medici, banker, 1427.
The results are in: ancestral earnings account for 10% of the total variation in earnings, and 17% of total variation in wealthy. Using a series of controls, you find a stable earnings elasticity of 0.04 - this means that if your ancestors 20 generations ago had earnings 1% higher, your earnings would rise by 0.04%. For wealth, this coefficient is between 0.02 and 0.03. In both cases the results are highly significant, which means that the chance of a false positive or false negative is extremely small - under 1% or 5% in basically all cases. Another way of visualizing the results is that, if your ancestors were at the top 10% (aka the 90th percentile) in 1427, instead of the bottom 10%, you’d earn 5% more; and if they jumped from the lowest to the highest decile, your wealth would be 12% higher. An interesting fact is that, by tertiles, the lowest classes have more or less equal possibilites of entering either the middle or upper third of the income distribution, but the richest 33% of the income scale has basically no risk of falling to the other two thirds.
These estimates seem very small, but this is over 400 years, and until that point it was believed that extremely distant ancestors might not have any benefit for present-day individuals. For example, a slightly different paper examining rare last names in England and Wales found an elasticity of 0.70-0.75 from 1858 to 2012, though this paper used wealth at death, which ignored pre-death gifts, sales, or bequests - significant for wealthy individuals. A paper that looks at the closeness of association among and between Swedish generations found significant persistence, but not due to genetic factors. Another study from Catalonia discovered that elasticity was of 0.60 for educational attainment, and explained it through assortative mating - i.e. the tendency of educated people to marry each other, and not the uneducated.
One first question is whether these results were contaminated by migration, surname disappearance, or simply the family itself dying out. If less successful families disappeared, or moved elsewhere in Italy, the results could be skewed; it could also be that Florence’s lack of economic mobility repelled migrants, meaning that places with more immigration have higher mobility and lower surname persistence. Comparing the distribution of wealth for 1467 last names versus all last names in 2011, they have roughly 6% higher median wealth than non-Renaissance families. This is a relevant caveat, but not a fatal one. Assuming that new last names have a persistence exactly equal to 0 (since they have no previous ancestors), this leaves the elasticity estimate at 0.016 for earnings (which is relevant at 10% significance) and 0.010 for wealth (relevant at 1%) - meaning that the original results were significantly weakened. A different regression, that also corrects for the probability of a larger family name surviving (note that last name popularity and wealth in 1427 are not correlated), finds similar results to the baseline.
Lastly, the persistence of professions is high: there is a positive and statistically significant correlation between having ancestors who were lawyers, bankers, and goldsmiths in 1427 and being one yourself in 2011, but not for doctors or pharmacists. This is in line with other estimates, finding that professions tend to run in families due to unobservables (such as culture, preferences, or skills) that are passed down from parents to children (though, until extremely recently, this was father to son).
To finish up, some links:
The original paper by Barone and Mocetti (ungated link)
A brief article by the authors discussing its main findings
Another paper about the impact of low socioeconomic mobility on Italy’s economy (fun fact: the first named author of this paper is named Maia)
A previous blog post about socioeconomic mobility and one about the economic lessons of Succession (the tv show)
Interestingly enough, while the top 10 most common last names only account for 4.9% of the US population, this figure is just 4.5% for Whites, 6.6% for Native Americans, around 13% for Black and Asian groups, and 16.3% for Hispanics.
For Black people, the surname density is probably linked to the historical and political significance of slavery and emancipation; meanwhile, Asian countries tend to have much more common last names, due to factors such as long historical records (meaning last names got lost centuries ago), or standardization of language.
Surnames in the Spanish-speaking world are also much more dominated by common names: the 10 most common last names in Argentina account for 10.4% of the population; of Chile’s 16.7 million people, 16.2 million had one of the most common 100 surnames; 24% of Mexicans had one of the top 10 most common names; and 54% of Spaniards had one of the top 25 last names in the country.